Tips Thanks Video transcript In this video were going to learn how to rationalize the denominator. Endgroup André Nicolas.
Rationalizing The Denominator Examples Videos Solutions Activities
Multiplying and dividing the rationalizing factor of denominator 3 13 1 3 1 3 1 3 1 2 3 2 1.
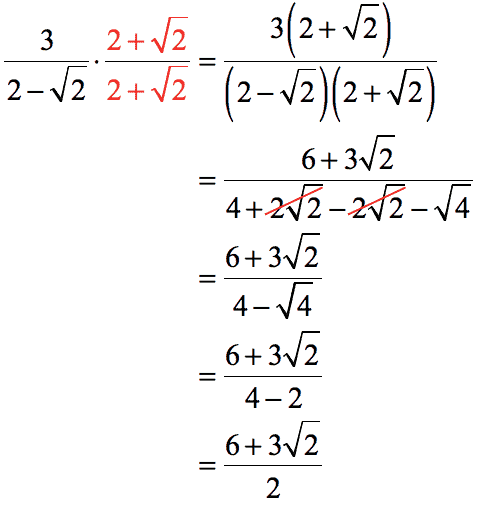
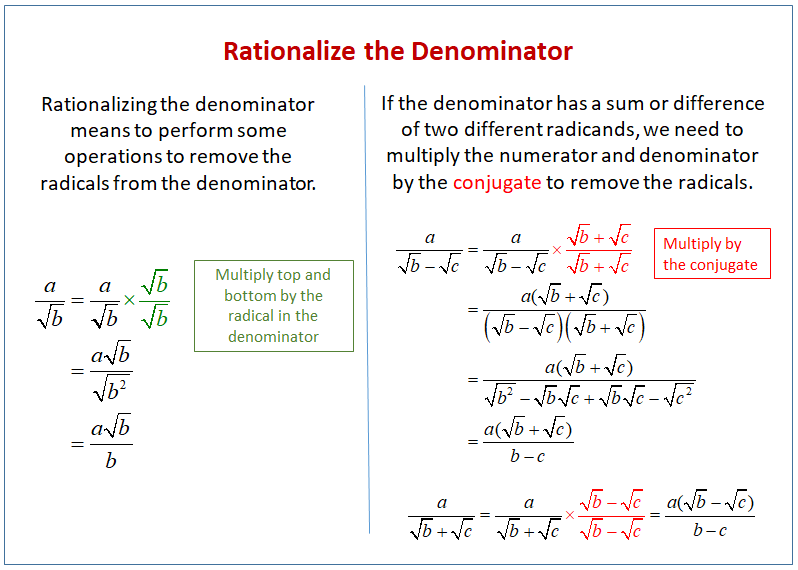
. To do this we have to multiply both the numerator and denominator by the root thats in the denominator. Whenever there is more than one term in the denominator rationalize it by multiplying by the conjugate. For example with a cube root multiply by a number that will give a cubic number such as 8 27 or 64.
Since the denominator is a binomial in which one of the terms is a square root we need to multiply the numerator and denominator by the conjugate of the binomial in. You can apply the same reasoning to rationalize a denominator which contains three terms. Web.
3 5 3 frac 3 5-sqrt 3 5 3 3. If the denominator is a binomial with a rational part and an irrational part then youll need to use the conjugate of the binomial. This multiplication doesnt change the overall value of the fraction as 7 7.
By the formula a b 2 a 2 2ab b 2. We can use this same technique to rationalize radical denominators. Instead to rationalize the denominator we multiply by a number that will yield a new term that can come out of the root.
How to Rationalize a Denominator with More Than One Term When there is more than one term under a radical sign in the denominator it becomes a bit trickier. This fraction has an irrational denominator 7 7 to rationalize we want to try and replace the 7 7 with a whole number. How do you rationalize the denominator of a radical expression that has two terms in the denominator.
Up to 10 cash back Rationalizing the Denominator by Multiplying by a Conjugate Rationalizing the denominator of a radical expression is a method used to eliminate radicals from a denominator. When a denominator has a higher root multiplying by the radicand will not remove the root. If you have more than just a single root in your denominator try conjugate method to.
Tex2 sqrt23 sqrt5tex is much messier to understand as a real number than. Multiply top and bottom by the square root of 2 because. For the three-sevenths fraction the denominator needed a factor of 5 so I multiplied by.
To rationalize multiply top and bottom by a_0a_1lambdaa_2lambda2a_0a_1mua_2mu2. It is ok to have an irrational number in the top numerator of a fraction. Full access to solution steps.
To do that we can multiply both the numerator and the denominator by the same root that will get rid of the root in the denominator. I could take a 3 out of the denominator of my radical fraction if. Rationalize the expression 3 13 1 In the expression rationalizing factor of denominator that would be 3 1.
Subscribe to get much more. The fraction has now been rationalized. Rationalize a Denominator Consider the fraction 2 7 7 2.
The difference of squares formula states that. To simplify you will need to multiply. For example we can multiply 12 by 22 to get 22.
Make an initial guess x_0. The historical reason for rationalizing the denominator is that before calculators were invented square roots had to be approximated by hand. You multiply the numerator and the denominator by the conjugate of the denominator.
Multiply the numerator and denominator by the radical in the denominator. There is a lot of simplification but it is still somewhat messy. 3 Simplify as needed.
This website uses cookies to ensure you get the best experience. I think the reason is that if the denominator is a rational number it is in a simpler form and fractions can then combine making operations more clear. Which is just 1.
A fraction with a monomial term in the denominator is the easiest to rationalize. 2 2 2. Both the top and bottom of the fraction must be multiplied by the same term because what you are really doing is multiplying by 1.
Rationalize the denominator. Now the denominator has a rational number 2. As you know if the denominator contains only two terms you could rationalize the denominator by multiplying the denominator by its conjugate.
To approximate sqrtn where n in mathbbN the ancient Babylonians used the following method. Denominator requires to multiply both the numerator and denominator of the fraction by a suitable number - usually the conjugate - so that when simplified the denominator is rational -. This can be done here by multiplying the top and bottom by 7 7 shown below.
Let x_k 1 fracx_k dfracnx_k2. 013 What we mean when we say rationalize the denominator Were basically just saying get the root out of the denominator. That way the roots will cancel.
A conjugate is the same expression but. A ba b a2 b2. Notebook Unlimited storage Personalized practice problems.
Frac 5 5 55. Created by Sal Khan and CK-12 Foundation. Free rationalize denominator calculator - rationalize denominator of radical and complex fractions step-by-step.

Radical In Denominator How To Rationalize The Denominator Video Lesson Transcript Study Com
To Rationalize A Denominator That Has More Than On Gauthmath
0 Comments